James W. Cannon and Warren
Dicks,
On hyperbolic once-punctured-torus
bundles II: fractal tessellations
of the plane.
Geom. Dedicata, 123 (2006), 11-63.
Subscribers to SpringerLink can download the article.
October 11, 2007 version, 60 pages, available as an 11Mb pdf .
We describe fractal tessellations of the plane that arise naturally
from Cannon-Thurston maps associated to complete, hyperbolic, once-punctured-torus
bundles. We determine the
symmetry groups of these tessellations. Thurston's drawings.
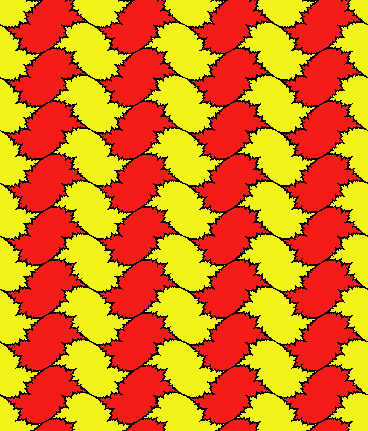 |
A fragment, depicted with L = yellow, R = red, of the Cannon-Thurston planar tessellation codified by
the bi-infinite word The tessellation has half-turn symmetries, vertical glide-reflection symmetries, and horizontal glide-reflection symmetries. The planar symmetry group is of type p g g. The quotient orbifold is a projective plane with two double points. |
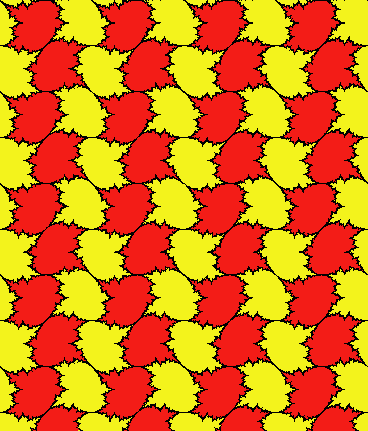 |
A fragment, depicted with L = yellow, R = red, of the Cannon-Thurston planar tessellation codified by
the bi-infinite word The tessellation has half-turn symmetries, vertical glide-reflection symmetries, and horizontal glide-reflection symmetries. The planar symmetry group is of type p g g. The quotient orbifold is a projective plane with two double points. |
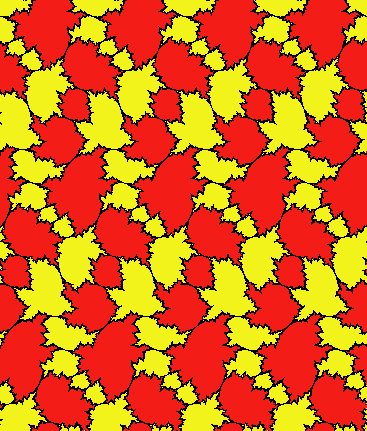 |
A fragment, depicted with L = yellow, R = red, of the Cannon-Thurston planar tessellation codified by
the bi-infinite word The tessellation has no half-turn symmetries and no glide-reflection symmetries. The planar symmetry group is of type p 1. The quotient orbifold is a torus. |
In Definition 7.3, the fourth and fifth sentences should be changed from
We then endow $\mathfrak{E}$ with the resulting lexicographic order on infinite reduced products of two-letter words. Thus two distinct ends $\mathcal{W}$, $\mathcal{V}$, are compared by finding the least $n \in \naturals$ such that $\mathcal{W}_{2n}^{-1}\mathcal{W}_{2n+2} \ne \mathcal{V}_{2n}^{-1}\mathcal{V}_{2n+2}$, and then comparing this pair of two-letter words.
to the single sentence
We then endow $\mathfrak{E}$ with the lexicographic order in which two distinct ends $\mathcal{W}$, $\mathcal{V}$, are compared by finding the least $n \in \naturals$ such that $\mathcal{W}_{n}^{-1}\mathcal{W}_{n+2} \ne \mathcal{V}_{n}^{-1}\mathcal{V}_{n+2}$, and then comparing this pair of two-letter words.
In Lemma 7.11 (ii)(1) "...(AC) = ..." should be "...(AC)D_{\mathcal{F}} = ..." twice.
More on the references:
[3] Bowditch, B.H.: The Cannon-Thurston map for punctured-surface groups. Math. Z. 255 (2007), 35-76.
[4] Cannon J.W., Thurston, W.P.: Group invariant Peano curves. Geom. Topol. 11 (2007), 1315-1355.
[10] Thurston, W.P.: Hyperbolic Structures on 3-manifolds, II: Surface groups and 3-manifolds which fiber over the circle. http://arXiv.org/abs/math/9801045
The tessellations studied in our article have two colors. Such tessellations are considered monochromatic
if the symmetry group is color-preserving, and are called dichromatic if the symmetry group contains a color-reversing element.
It is well known that there are exactly 17 types of monochromatic tessellation of the plane. In our article, exactly
2 of the 17 types appear, namely p1 and p2. We have learned from Marcus du Sautoy that there are exactly
46 types of dichromatic tessellation of the plane and that this was first proved in
H. J. Woods, Counterchange symmetry in plane patterns, Journal of the Textile Institute, 27 (1936), T305-T320;
see
H. S. M Coxeter, Coloured symmetry, pp. 15-33 in
M.C. Escher, Art and Science (eds. H.S.M. Coxeter, M. Emmer, R. Penrose, and M. L. Teuder), Elsevier, Amsterdam, 1986.
In our article, exactly 2 of the 46 types appear, namely pg/p1 and pgg/p2. Coxeter asserts that
among M. C. Escher's 137 Symmetry Drawings examples can be found of exactly 14 of the 46 types, with
Symmetry Drawing 67 being of type pg/p1 and Symmetry Drawing 39 being of type pgg/p2; see pages 169 and 146 of
Doris Schattschneider, Visions of symmetry: notebooks, periodic drawings and related work of M. C. Escher,
W. H. Freeman, New York, 1990.
Up to Warren
Dicks' publications.